Cosmology is the study of the origin and evolution of the cosmos, where by cosmos we mean everything – no less. This is a subject which has always fascinated mankind and has given rise to many fanciful and often farcical stories. It is one of the principle reasons for the invention of religion. We looked at one such story in the introduction, the cyclic creation, maintenance and destruction of the cosmos by Brahma, Vishnu and Shiva. Now let’s get serious.
What we know about the Big Bang
We know from astronomical observation that the universe – space – is expanding. The stars, galaxies and other distant celestial bodies are moving away from us (and we, from them). Observation of type 1a supernovae (explained later) has shown that for the last 7 Gy the expansion has been accelerating slowly.
If we do a backwards extrapolation of the measured expansion, we find that about 14 Gya all spatial objects were in the same place. This figure has recently been refined to 13.8 Gya.[ref]According to the WMAP project, the age of the universe is 13.77 ± 0.059 billion years. http://map.gsfc.nasa.gov/universe/uni_age.html. The ESA Planck probe finds 13.799 ± 0,021 billion years. We will settle for 13.8 Gya.[/ref] The entire visible universe once occupied a very small region, smaller than the size of an atom.
It is important to understand that we can see only part of the cosmos, the visible universe, or what we call “our Universe”. It is limited in size because of the time light takes to get from a distant point to us and because of the age of the universe. Light travels with a finite speed, so the more distant an object or event, the more time light takes to reach us and the farther back in the past the event we are seeing. The most distant objects we can see are therefore the oldest, those which emitted their light at the time of the Big Bang, about 14 Gya. Because space has been expanding in the meantime, such objects are now about 46 Giga light-years away, which is thus the current radius of the visible universe.[ref]Carroll (2010), 387n38.[/ref] It is, of course, getting bigger all the time.
Current hypothesis – inflation
Astronomers, cosmologists and physicists – the folks who worry about this sort of thing — generally accept the Inflationary Big Bang as the explanation of the origin of our universe, simply because it is capable of accounting for many facts left unexplained by the original, non-inflationary Big Bang hypothesis.[ref]Some scientists contest inflation, even hotly. See, e.g., Hossenfelder, “Is the inflationary universe a scientific theory? Not anymore.”. http://backreaction.blogspot.fr/2017/10/is-inflationary-universe-scientific.html[/ref]
The inflationary Big Bang framework says that the universe has evolved in a two-step process:
- a brief and extremely rapid expansion, called inflation, followed by
- subsequent, slower expansion powered by the negative pressure of gravity (the cosmological constant).
In a nutshell, our universe started out smaller than the current size of an atom. It was roughly uniform but with tiny quantum-mechanical fluctuations (often referred to as “jitters”). Almost instantly, on a human time scale, it inflated (expanded) enormously to about the size of a grapefruit.[ref]Tegmark (2014), 117.[/ref] The rapid expansion isolated the quantum jitters before they could resolve. When inflation stopped, a mainly uniform space had been filled with matter formed from the spread-out quantum fluctuations. Since that time, it has expanded well beyond what we can see of it.
It must be stressed that when we speak of the expansion of space, we mean the “fabric” of space itself. The expansion is a property of space only: As space expands, the push to expand remains the same at each (new) point and does not dilute away. Only space expands and only on very large scales. On smaller scales, the other physical forces, such as gravity, keep particles and planets together. Although the space around our galaxy, the Milky Way, is expanding, the galaxy is not. Nor are the stars, nor the planets, nor you nor I.[ref]If you are expanding, you cannot blame it on universal inflation.[/ref]
One of the strong points of the inflationary Big Bang is that it explains how the expansion started. It was due to the properties of the inflaton field. Read on.
The origin of inflation is thought to be due to a field called the inflaton field (or false vacuum). This field was originally in a metastable state of high energy in which it exerted a huge outwardly-directed force which caused space to expand enormously, in fact exponentially, doubling its size every 10-37 seconds.
Such an increase in size rapidly becomes huge. At that scale, doubling its size every 10-37 seconds, one hundred doublings would have taken about 10‑35 seconds, but would have resulted in a total increase by a factor of 2100 = 1.3×1030.[ref]Greene (2004), 308, Guth (1997), 173. Max Tegmark says the doubling may have occurred every 10‑38 seconds.[/ref]
Here’s more detail. The inflaton field was in a state of unstable equilibrium. Anyone who has ever been delicately balanced, say on a taut rope, so that if she leans one way or the other she starts to fall, understands what it means to be in unstable equilibrium. At about 10-35 seconds of age[ref]Greene (2004), 285.[/ref], the field “lost its balance” and started to “fall” (metaphorically) off its high value. After about 10-32 seconds, space had “fallen” as far as it could in the inflaton field, effectively hitting “bottom”. More precisely, it reached its point of minimum energy in the inflaton field. The inflation stopped but the universe went on expanding, but more slowly, under the outwardly-directed pressure of gravity (the cosmological constant). The energy released by the “falling” inflaton field provided the energy and matter that constitute the universe today. As each bit of matter exerted a gravitational attraction on every other bit, the rate of expansion slowed down. When the universe attained the age of about 7 billion years, the expansion rate began accelerating. More on that later.
At its pre-inflation size, the universe was so small that it must be explained using quantum mechanics, according to which states of energy normally forbidden by the Law of Conservation of Energy can exist for very short periods of time, as explained in the theory chapter. So there were a great number of relatively very tiny places where the energy was higher or lower than the average. The rapid inflationary expansion caught these energy fluctuations unaware, so to speak, and scattered them out over macroscopic scales before they could merge and even out the energy. They gave rise to particles and antiparticles. This tiny non-uniformity has been observed in the cosmic microwave background radiation (explained shortly).
The inflationary Big Bang solves the so-called horizon problem: How the universe managed to reach large-scale uniformity without there having been enough time for light to traverse it. Before the inflaton field started “falling”, the universe was so tiny that light could travel from any part to any other, so it managed to reach a state of internal equilibrium in which it was all at practically the same temperature everywhere. As the universe inflated, this region of almost constant temperature did too, so the universe we see today is approximately homogeneous, at least on a large scale, a fact which is confirmed by satellite observation.[ref]NASA WMAP project, http://map.gsfc.nasa.gov/universe/bb_cosmo_fluct.html. These results have been refined by the more recent Planck probe.[/ref]
Inflation also resolves the so-called flatness problem, since it was so fast that the resulting universe looks flat. (Think of the surface of a balloon which is rapidly inflated to a very large size.[ref]Better yet, glue some 2-centime pieces to the balloon, before you inflate it more. You will see how the coins move apart without getting any larger.[/ref]) In cosmology, flatness is very important, as it means the expansion will continue; otherwise, the universe would begin to collapse back together, terminating in a “Big Crunch”.[ref]The flatness problem is more complex than this says, but it gives the idea. [/ref](More on flatness late, too.)
There are several reasons to think inflationary exponential expansion took place. It explains why expansion took place (the inflaton field), as well as why space has a uniform temperature everywhere on the average. And it shows how perfectly “normal” quantum-mechanical phenomena (briefly-existing spots of non-conserved energy) gave rise to packets of energy and eventually particles.
This much is agreed on by almost all versions of the inflationary Big Bang theory, but there are several versions of it — far too many, according to critics.
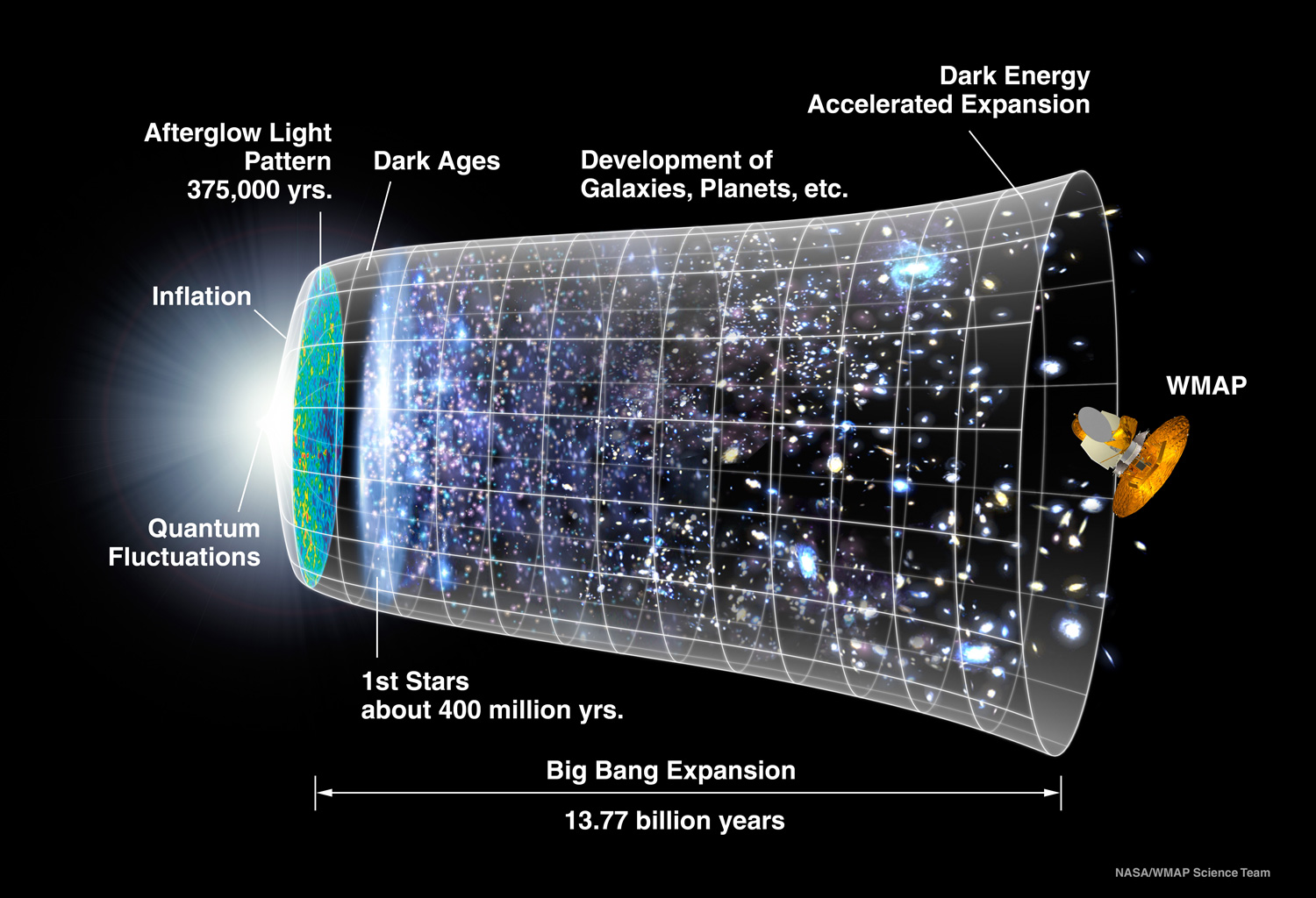
Time line of the inflationary Big Bang, from WMAP via NASA
Infinite expansion and the inflationary multiverse
No one knows if the universe is infinite. Period.
There are many versions of inflation. In some models, expansion goes on forever. In small, local regions, the inflaton field may decay non-uniformly into “bubbles” (like boiling water). Each bubble may fall off its metastable energy peak to give up energy to form … a universe! Each one looks like ours to the extent that it has been born out of an inflationary burst, but the physics may vary from one to another. (This process has been compared to cell divisio[ref]Guth (1997), 251.[/ref], but does this suggest that the inflationary multiverse should be regarded as an evolutionarily evolving population of universes? I think not.)
Such universes within the inflationary multiverse have been called “pocket” or “bubble” universes. Each one is infinite. Although the pocket may seem finite from the outside, time is transformed into space inside due to the effects of relativity, making the interior infinite!
Since inflation continues forever in this scenario, the Big Bang at the origin of our universe was really the moment when inflation in our part of the multiverse stopped.
This multiverse hypothesis is a suggestion on the extreme edge of cosmology, but it is taken seriously by many cosmologists. In fact, there are other multiverses proposed. For more info, see the multiverse page.
L’après Big Bang — nucleosynthesis and background radiation
Once the inflaton field’s energy was spent, inflationary expansion ended. The universe went on growing under the pressure of the cosmological constant, but the gravitational attraction of all that mass limited the expansion. As the universe expanded, it cooled.[ref]Think of how a bicycle tire gets hot as you pump air into it; this is just the opposite phenomenon. All temperatures from Guth (1997), 86-94.[/ref] It was then that the first step in the formation of chemical elements began.
| Time after Big Bang |
Temperature of universe[ref]All temperatures from Guth (1997), 86-94.[/ref] |
| 10‑39 secs |
1020K |
| 1 sec |
1010K |
| 7 days |
17×106K |
| 1 year |
2×106K |
| 100 Kyrs |
5.8×103K |
Big-Bang nucleosynthesis, the formation of the light chemical elements, hydrogen, helium and lithium, took place in the period from about 1 sec (or less) to 3-4 minutes after the Big Bang. Hee’s how.
At .1 sec ABB, it was too hot for atomic nuclei to form. The universe was filled with rapidly moving protons and neutrons, electrons and positrons, neutrinos and antineutrinos and lots of radiation (photons). Protons and neutrons constantly changed into one another.[ref]The ratio of protons to neutrons was 1.61, due to the equilibrium rate of the reactions.[/ref]
Remember the four basic forces of physics? At such high temperatures, their impact was negligible. But as temperatures fell and particles moved more slowly, the strong force was able to exert its influence – by forming nuclei (but not yet atoms).
At around one second of age[ref]Guth (1997), 94.[/ref], the universe’s temperature was “cool” enough that the frenetic activity of all those particles calmed some. Protons stopped changing into neutrons, but neutrons still decayed into protons and electrons.[ref]Since neutrons are slightly heavier than protons.[/ref] Neutons which did not decay combined to make an isotope of hydrogen called deuterium (2H, one proton + one neutron). Electrons and positrons annihilated to form huge amounts of photons, visible today as the Cosmic Microwave Background radiation. By 30 seconds of age, about half the electrons and positrons had mutually annihilated.
At about 3 minutes of age, the universe became an element-producing nuclear furnace. Deuterium nuclei combined to form helium (4He). In this way, the lighter elements, including small amounts of lithium (3Li), were formed. This was the era of Big-Bang nucleosynthesis, the first of three steps in the formation of the elements of the universe. At the end of this period, there were seven protons for every neutron. Free neutrons mostly combine with protons to make 4He, so the Universe was composed of 75% protons and 25% 4He by mass and still is today, give or take a small amount of trace elements.[ref]Take 14 protons and 2 neutrons, make as much He as possible, and you get 12 protons (total atomic mass ~12) and one He (atomic mass ~4), which gives the 75:25 ratio.[/ref]
The Cosmic Microwave Background Radiation (CMB)
As the universe continued expanding and cooling, more heat was given off in the form of radiation, i.e., photons. The photons had such high energy that they continually knocked electrons loose from any protons they might bond with, keeping all the atoms ionized. So the matter was in the form of a plasma, a “soup” of rapidly-moving free protons, neutrons and electrons. Just as the photons released electrons from atoms, so the electrons scattered the photons, which were thus like light in a cloud or fog. In a word, the universe was opaque.
After about 380,000 years, the temperature of the universe descended to around 3000 Kelvin, and the electromagnetic force bound electrons with nuclei to form atoms, a process called recombination by cosmologists. The binding of electrons in atoms was equivalent to a phase change (like, say, water freezing to ice) and is considered as such by physicists. Photons now were no longer deflected by electrons and the universe became transparent, although the number of photons was so low that it was still dark and there were not yet any stars to make more light. The photon radiation was emitted in all directions and is still “visible” today.[ref]Since it is in the microwave frequency, we cannot really see it. [/ref] This Cosmic Microwave Background Radiation (CMB) is light from 13.3 Gya.
When we look up into the sky, we are looking into the past, because of the time light from distant celestial objects takes to reach us. But because the universe was opaque until 380 Ky ABB, we can see no farther back than that. The most distant light we can detect is composed of the remnants of radiation emitted then, now reduced now reduced by the expansion of space to microwave frequencies.
Here’s a nit: Recombination took place over a short period of time. Shortly after its beginning but before it finished, enough electrons were bound that photons could begin to move about without scattering. So this latter event, called decoupling of photons, took place a little after recombination.
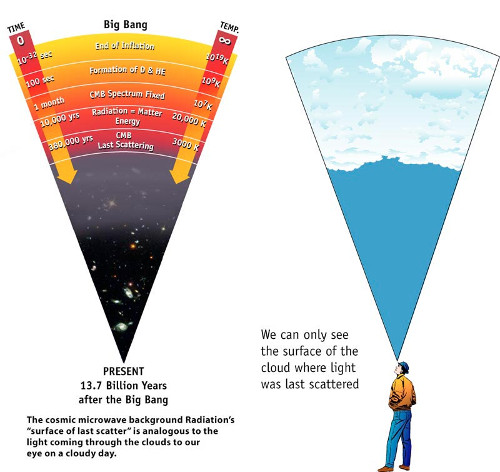
Just as we cannot see past the surface of a cloud, we cannot see past the time when the universe became translucid. From NASA
The great uniformity in the distribution of this radiation lends support to the cosmological principle, the idea that the universe is uniform or homogeneous on very large scales, on the order of 106 light-years.
After about 200My, something happened to dispel the darkness. To find out what, read about the life of stars.










![Phylogenetic tree By MPF [Public domain], via Wikimedia Commons](http://natural-universe.net/wp-content/uploads/2015/07/800px-PhylogeneticTree.png)